This report was originally published 11 May 2017.

Summary

In recent years, participation in Science, Technology, Engineering and Mathematics (STEM) subjects has garnered increased public focus and worldwide coverage. In Australia, the contribution that STEM makes to the economy is substantial, with the advanced physical and mathematical sciences sector currently estimated to contribute over 22 per cent of Australia’s economic activity, adding approximately $292 billion per year in gross value-added to the economy (Australian Academy of Science 2015). The importance of innovation and technology to the world economy has also shifted government and schools’ priorities to place a greater emphasis on STEM through education policy. For example, the Australian federal government recently announced $112 million in spending to boost engagement in STEM among young Australians (Commonwealth of Australia 2016).

Despite the increased focus on STEM in education, student enrolments in fundamental STEM subjects such as mathematics that includes calculus have decreased over the last few decades in Australia (Board of Studies Teaching and Educational Standards NSW 2016a; Dekkers & de Laeter 2001; Lyons & Quinn 2015). In New South Wales (NSW), overall enrolments in mathematics in the Higher School Certificate (HSC) have increased by seven per cent from 2001 to 2015. However, enrolments in the HSC Mathematics course, an intermediate mathematics course that includes calculus, decreased by 4,453 enrolments over this period (see Figure 1 below). This reflects a drop from 39 per cent of all mathematics enrolments in the HSC in 2001 to 29 per cent in 2015. At the same time, enrolments in the HSC General Mathematics course (does not include calculus; renamed Mathematics General 2 in 2014) have increased by nine per cent since 2001. These enrolment trends suggest that there has been a shift from HSC Mathematics towards HSC General Mathematics, which may have implications for a future where STEM skills are increasingly important.

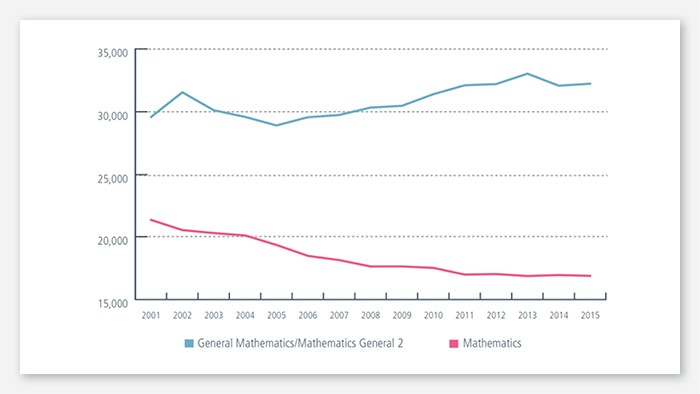
These trends in mathematics enrolments have led to questions about the university admission processes, in particular the scaling of the Australian Tertiary Admissions Rank (ATAR) and the lack of pre-requisites for studying tertiary STEM subjects. In a survey of NSW mathematics teachers, 51 per cent reported believing that students were picking Year 12 mathematics subjects below their ability, with a number of respondents stating that students were doing so to maximise their ATARs¹ (Mathematical Association of New South Wales 2014). This view was also echoed in a survey of university students who were required to take mathematics bridging courses because they did not study mathematics at a sufficiently advanced level in high school. Among the surveyed students, 45 per cent² reported maximising ATAR as a major reason for not studying higher levels of mathematics in school (Gordon & Nicholas 2013).

Policymakers have also publicly criticised the impact of the university admission processes on decreasing STEM enrolments and are proposing policy solutions to arrest these trends. Some have proposed building on “aspiration rather than compulsion” (Association of Heads of Independent Schools of Australia 2015, p. 2), such as the Council of Australian Governments’ (COAG) Education Council recommending the use of university entrance bonus point schemes for studying mathematics and advanced STEM subjects in schools (COAG Education Council 2015). Others have suggested making STEM study in school mandatory for progression to tertiary STEM studies through the reintroduction of a pre-requisite of at least Year 12 intermediate mathematics (i.e. HSC Mathematics) for all bachelor programs in science, engineering and commerce (Australian Academy of Science 2016). Recently, the Board of Studies Teaching and Educational Standards (BOSTES) NSW, now known as the NSW Education Standards Authority (NESA), announced new draft reforms that introduce new common content and marking scales between HSC General Mathematics and HSC Mathematics to “provide a disincentive to students choosing lower-level courses to gain a perceived advantage in their ATAR” (Board of Studies Teaching and Educational Standards NSW 2016b, p. 18). These reforms are set to be implemented from 2019.

Two factors that may be associated with the apparent link between taking the HSC General Mathematics course and maximising ATAR are student demographics and subject offerings in high school. Both of these factors have changed substantially since scaling was first introduced in NSW with the 1964 implementation of quotas on university places (Universities Admissions Centre 2015). Across Australia, retention rates to Year 12 drastically increased in the 1980s, from only 35 per cent in 1982 to 77 per cent in 1992 (Ainley, Kos & Nicholas 2008). This led to more diversity in Year 12 cohorts in terms of student characteristics (e.g. wider range of academic ability and increased proportion of female students) and in terms of subject preferences (Dekkers & de Laeter 2001). In response to this increased diversity, subject offerings in high schools began to expand in the 1980s, giving students more choice in their study options (Dekkers & de Laeter 2001). This increase in course diversity, combined with the elimination of pre-requisites for university STEM courses, may have made it less likely that students would choose to study higher level science and mathematics in Year 12. Indeed, if these subjects are no longer necessary to gain acceptance to a tertiary STEM degree, students may choose alternatives that better fit their preferences. This suggests that wider diversity in both student preferences and high school subject offerings are likely contributing factors to the reduction in enrolments in STEM subjects observed across Australia (Lyons & Quinn 2015).

Although much of the current scaling process was in place before these developments, these factors are still relevant to a situation where HSC General Mathematics students, on average, achieve higher scaled marks in mathematics than similar HSC Mathematics students, herein defined as a scaling advantage for HSC General Mathematics. Scaling is used to compare the marks of groups of students that study different subjects to account for the fact that a student’s mark and rank in a particular subject is dependent on both their ability and the abilities of others who study the same subject (Universities Admissions Centre 2015). Scaling achieves this through a system of equations that equates the scaled mean of all students who studied a subject (for example HSC Mathematics) to the scaled means those same students achieved in all their other subjects (Universities Admissions Centre 2015). This raises scaled marks for a subject if the students who studied HSC Mathematics performed better in their other subjects relative to the students who did not study HSC Mathematics. This means that scaling is dependent on the overall subject choices made by students with differing ability and is therefore vulnerable to changes in the preferences that drive these choices.

Recent research indicates that there may be a scaling advantage for HSC General Mathematics over HSC Mathematics. Pitt (2015) examined results for all students in NSW who studied either HSC General Mathematics or HSC Mathematics in 2013 and compared their Year 12 mathematics scaled marks and their Year 10 School Certificate (SC) scores in mathematics from 2011. Results showed that for students with similar Year 10 SC scores, those who chose HSC General Mathematics achieved higher scaled marks than those who chose HSC Mathematics, demonstrating a significant scaling advantage for HSC General Mathematics³. Despite mathematics that includes calculus (e.g. HSC Mathematics) being essential to tertiary STEM studies, the competition for university places and the need to achieve a high ATAR may drive many students who want to pursue STEM studies to choose mathematics that does not include calculus (e.g. HSC General Mathematics) in order to maximise their ATAR (Gordon & Nicholas 2013). The existence of a scaling advantage combined with the additional challenge of studying HSC Mathematics (Gordon & Nicholas 2013; Mathematical Association of New South Wales 2014) may be persuasive factors in leading students to choose the HSC General Mathematics course.

Aims

The objective of this research was to examine whether the current scaling process⁴ used in NSW provides a disincentive for students to study HSC Mathematics. Specifically, the current study aimed to address four key research questions:

1. Has there been a scaling advantage for HSC General Mathematics over HSC Mathematics for the years 2009 to 2013?
2. Of the student and school characteristics available, which student- and school-level characteristics are related to student choice of HSC General Mathematics rather than HSC Mathematics?
3. Do students studying STEM subjects at university regret choosing HSC General Mathematics?
4. Does the perceived scaling advantage or subject workload have greater influence on choosing HSC General Mathematics over HSC Mathematics?

The current study aimed to re-examine the results reported by Pitt (2015) by using a more rigorous method of comparing outcomes for matched students with similar characteristics (described in further detail in the Method section). The current study also extends these earlier findings by investigating scaling outcomes across five years and by examining the characteristics and factors associated with students’ subject choices as well as their views on their course choice once at university. However, the current study does not examine scaling in relation to the Mathematics Extension subjects nor does it cover the full breadth of characteristics and factors that impact subject choice such as students’ interests.